Exit-Problem for a Class of Non-Markov Processes With Path Dependency

The main result of the paper states that, under some assumptions on potentials V and F, and on domain G, Kramers’ type law for the exit-time holds.


This content originally appeared on HackerNoon and was authored by Class Path

Abstract and 1 Introduction

1.1 State of the art

1.2 Some remarks on dynamics and initial condition

1.3 Outline of the paper

1.4 List of notations

2 Large Deviation Principle

2.1 Establishing the LDP for the SID

2.2 Results related to the LDP

2.3 Compactness results

3 Exit-time

3.1 Auxiliary results

3.2 Proof of the main theorem

3.3 Proofs of auxiliary lemmas

4 Generalization and References

Abstract

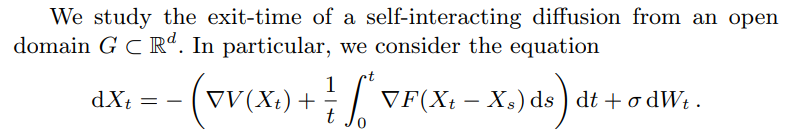
\ We are interested in small-noise ( σ → 0) behaviour of exit-time from the potentials’ domain of attraction. In this work rather weak assumptions on potentials V and F, and on domain G are considered. In particular, we do not assume V nor F to be either convex or concave, which covers a wide range of self-attracting and self-avoiding stochastic processes possibly moving in a complex multi-well landscape. The Large Deviation Principle for Self-interacting diffusion with generalized initial conditions is established. The main result of the paper states that, under some assumptions on potentials V and F, and on domain G, Kramers’ type law for the exit-time holds. Finally, we provide a result concerning the exit-location of the diffusion.

1 Introduction

\ \

\

\

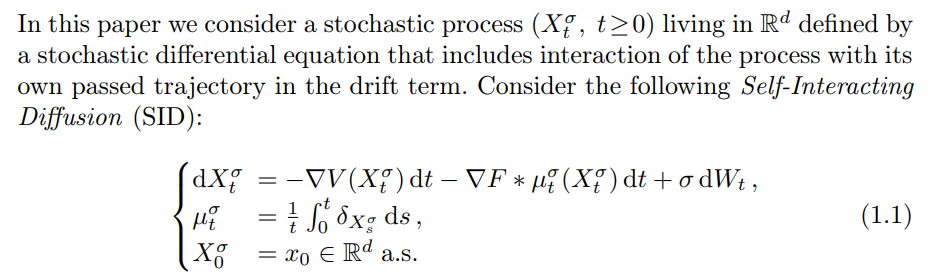
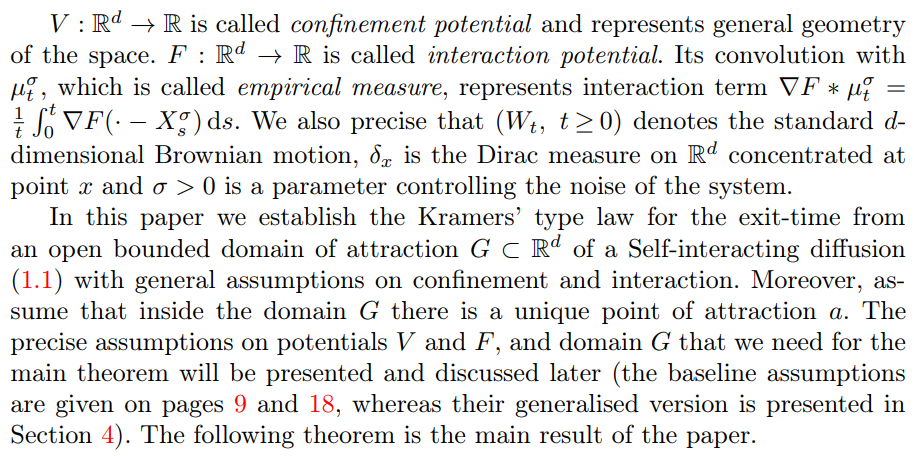
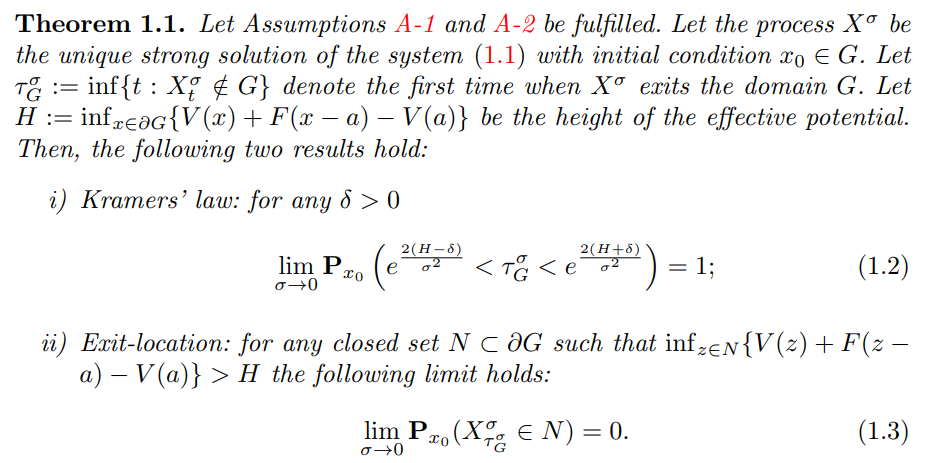
:::info This paper is available on arxiv under CC BY-SA 4.0 DEED license.

:::

:::info Authors:

(1) Ashot Aleksian, Université Jean Monnet, Institut Camille Jordan, 23, rue du docteur Paul Michelon, CS 82301, 42023 Saint-Étienne Cedex 2, France;

(2) Aline Kurtzmann, Université de Lorraine, CNRS, Institut Elie Cartan de Lorraine UMR 7502, Vandoeuvre-lès-Nancy, F-54506, France;

(3) Julian Tugaut, Université Jean Monnet, Institut Camille Jordan, 23, rue du docteur Paul Michelon, CS 82301, 42023 Saint-Étienne Cedex 2, France.

:::

\


This content originally appeared on HackerNoon and was authored by Class Path


Print Share Comment Cite Upload Translate Updates
APA

Class Path | Sciencx (2025-03-01T02:46:56+00:00) Exit-Problem for a Class of Non-Markov Processes With Path Dependency. Retrieved from https://www.scien.cx/2025/03/01/exit-problem-for-a-class-of-non-markov-processes-with-path-dependency/

MLA
" » Exit-Problem for a Class of Non-Markov Processes With Path Dependency." Class Path | Sciencx - Saturday March 1, 2025, https://www.scien.cx/2025/03/01/exit-problem-for-a-class-of-non-markov-processes-with-path-dependency/
HARVARD
Class Path | Sciencx Saturday March 1, 2025 » Exit-Problem for a Class of Non-Markov Processes With Path Dependency., viewed ,<https://www.scien.cx/2025/03/01/exit-problem-for-a-class-of-non-markov-processes-with-path-dependency/>
VANCOUVER
Class Path | Sciencx - » Exit-Problem for a Class of Non-Markov Processes With Path Dependency. [Internet]. [Accessed ]. Available from: https://www.scien.cx/2025/03/01/exit-problem-for-a-class-of-non-markov-processes-with-path-dependency/
CHICAGO
" » Exit-Problem for a Class of Non-Markov Processes With Path Dependency." Class Path | Sciencx - Accessed . https://www.scien.cx/2025/03/01/exit-problem-for-a-class-of-non-markov-processes-with-path-dependency/
IEEE
" » Exit-Problem for a Class of Non-Markov Processes With Path Dependency." Class Path | Sciencx [Online]. Available: https://www.scien.cx/2025/03/01/exit-problem-for-a-class-of-non-markov-processes-with-path-dependency/. [Accessed: ]
rf:citation
» Exit-Problem for a Class of Non-Markov Processes With Path Dependency | Class Path | Sciencx | https://www.scien.cx/2025/03/01/exit-problem-for-a-class-of-non-markov-processes-with-path-dependency/ |

Please log in to upload a file.



There are no updates yet.
Click the Upload button above to add an update.

You must be logged in to translate posts. Please log in or register.

Related Posts