This content originally appeared on HackerNoon and was authored by Labyrinthine
Table of Links
1.1 A Polyethylene-based metamaterial for acoustic control
2 Relaxed micromorphic modelling of finite-size metamaterials
2.1 Tetragonal Symmetry / Shape of elastic tensors (in Voigt notation)
4 New considerations on the relaxed micromorphic parameters
4.2 Consistency of the relaxed micromorphic model with respect to a change in the unit cell’s size
4.3 Relaxed micromorphic cut-offs
6 Fitting of the relaxed micromorphic parameters with curvature (with Curl P)
6.1 Asymptotes and 6.2 Fitting
8 Summary of the obtained results
9 Conclusion and perspectives, Acknowledgements, and References
A Most general 4th order tensor belonging to the tetragonal symmetry class
B Coefficients for the dispersion curves without Curl P
C Coefficients for the dispersion curves with P
D Coefficients for the dispersion curves with P◦
A Most general 4th order tensor belonging to the tetragonal symmetry class
Considering the following quadratic form
\

\ where L is a 4th order tensor and D is a 2nd order one, the most general form of L if it belongs to the tetragonal symmetry class written in Voigt notation is
\
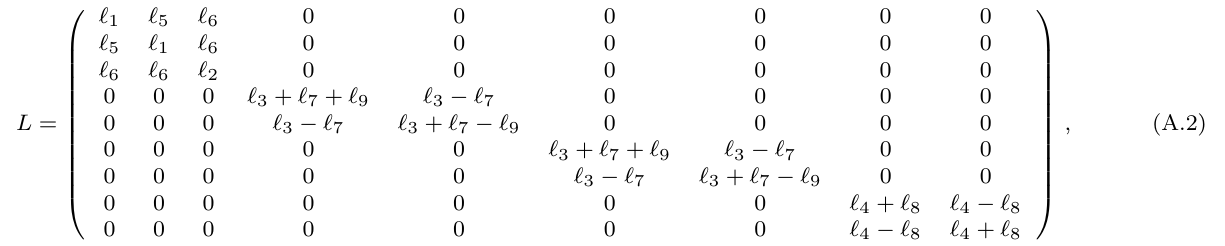
\ where the order of the element of the vector associated with the quadratic form A.1 is
\
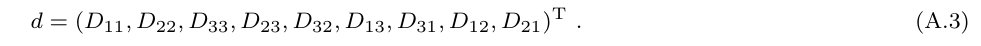
If we now split the tensor D in its symmetric and skew-symmetric part, the corresponding vector in Voigt notation are
\
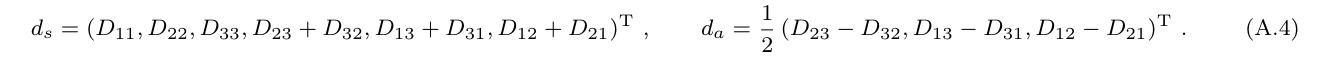
\ Because of the class of symmetry considered, it is necessary to take into account a mixed constitutive matrix that couples the symmetric and skew-symmetric part of D in order to build back the quadratic form Y
\
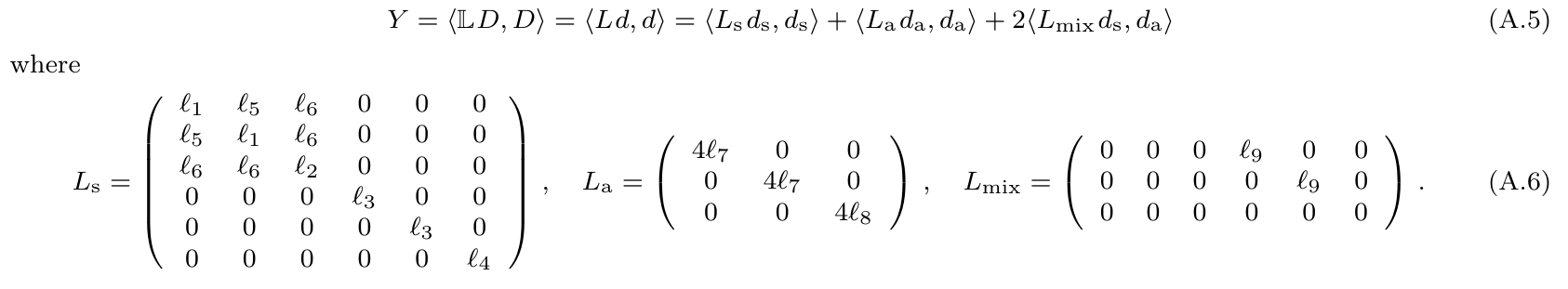
\
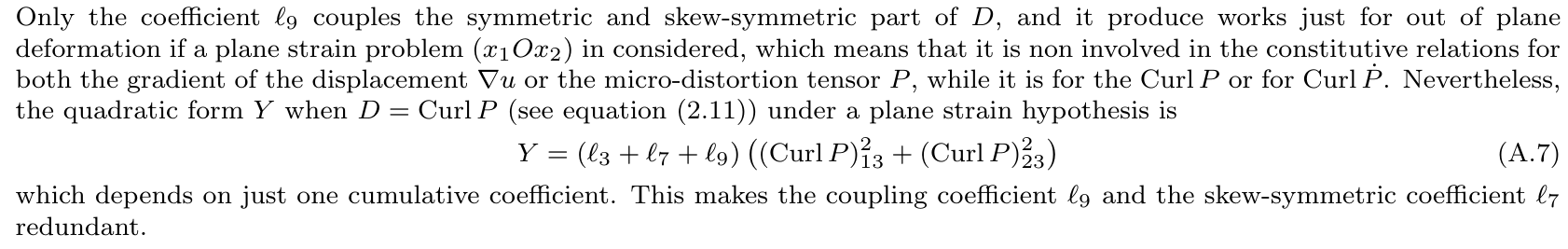
B Coefficients for the dispersion curves without Curl P
\

\
C Coefficients for the dispersion curves with Curl P
\
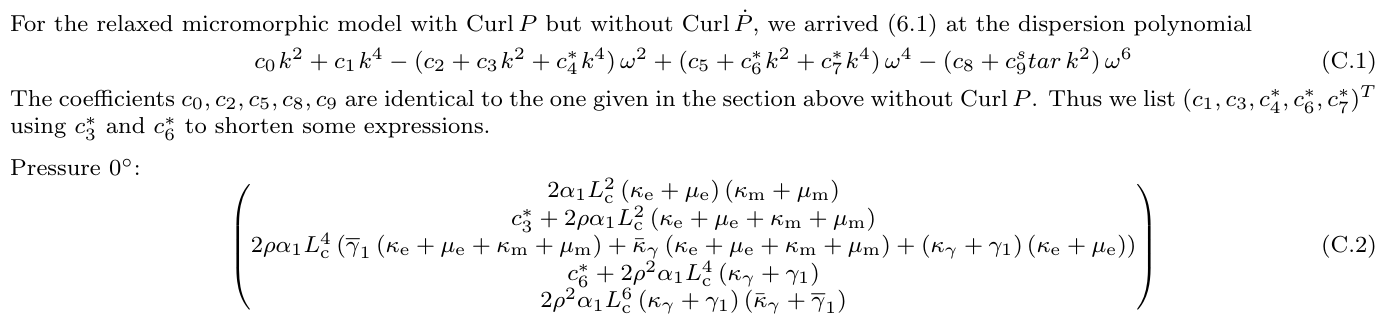
\
\
\
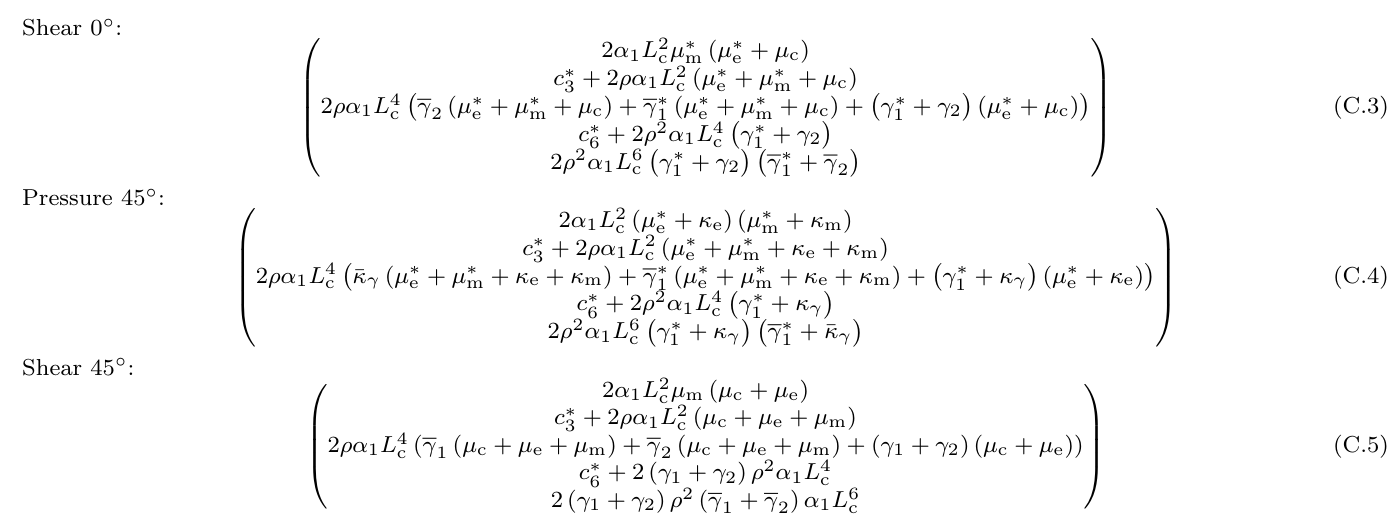
\
\
\
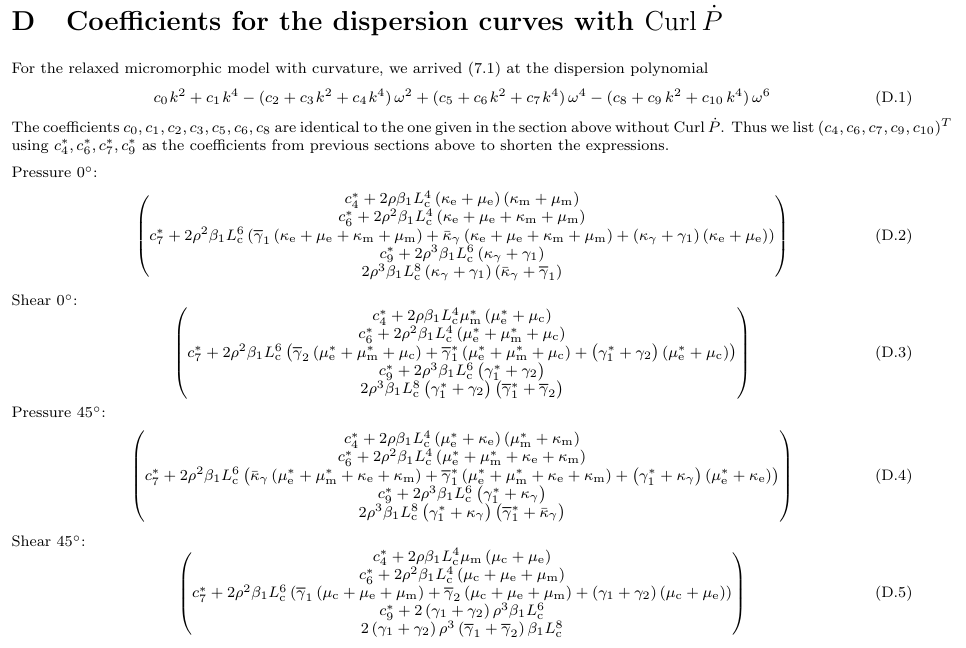
\ \
:::info This paper is available on arxiv under CC BY 4.0 DEED license.
:::
:::info Authors:
(1) Jendrik Voss, Institute for Structural Mechanics and Dynamics, Technical University Dortmund and a Corresponding Author (jendrik.voss@tu-dortmund.de);
(2) Gianluca Rizzi, Institute for Structural Mechanics and Dynamics, Technical University Dortmund;
(3) Patrizio Neff, Chair for Nonlinear Analysis and Modeling, Faculty of Mathematics, University of Duisburg-Essen;
(4) Angela Madeo, Institute for Structural Mechanics and Dynamics, Technical University Dortmund.
:::
\
This content originally appeared on HackerNoon and was authored by Labyrinthine
Labyrinthine | Sciencx (2025-03-23T17:31:13+00:00) How Symmetric and Skew-Symmetric Tensors Interact. Retrieved from https://www.scien.cx/2025/03/23/how-symmetric-and-skew-symmetric-tensors-interact/
Please log in to upload a file.
There are no updates yet.
Click the Upload button above to add an update.
